The design process of modern buildings and structures is regulated by a huge number of different building codes and regulations. In most cases, standards require certain characteristics to be ensured, for example, deformation or deflection of floor slab beams under static or dynamic load. For example, SNiP No. 2.09.03-85 determines for supports and overpasses the deflection of the beam is no more than 1/150 of the span length. For attic floors this figure is already 1/200, and for interfloor beams it is even less - 1/250. Therefore, one of the mandatory design stages is to perform a beam deflection calculation.

Ways to perform deflection calculations and tests

The reason why SNiPs establish such draconian restrictions is simple and obvious. The smaller the deformation, the greater the margin of strength and flexibility of the structure. For deflection less than 0.5% load-bearing element, the beam or slab still retains elastic properties, which guarantees normal redistribution of forces and maintaining the integrity of the entire structure. As the deflection increases, the building frame bends, resists, but stands; when the permissible value is exceeded, the bonds break, and the structure loses its rigidity and load-bearing capacity like an avalanche.

• Use an online software calculator, in which standard conditions are “hardwired”, and nothing more;
• Use ready-made reference data for various types and types of beams, for various support load patterns. It is only necessary to correctly identify the type and size of the beam and determine the desired deflection;
• Calculate the permissible deflection with your hands and your head; most designers do this, while controlling architectural and construction inspectors prefer the second method of calculation.

For your information! To really understand why it is so important to know the magnitude of the deviation from the initial position, it is worth understanding that measuring the amount of deflection is the only accessible and reliable way to determine the condition of the beam in practice.

By measuring how much the ceiling beam has sagged, you can determine with 99% certainty whether the structure is in disrepair or not.

Method of performing deflection calculations

Before starting the calculation, you will need to remember some dependencies from the theory of strength of materials and draw up a calculation diagram. Depending on how correctly the diagram is executed and the loading conditions are taken into account, the accuracy and correctness of the calculation will depend.

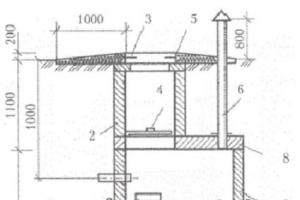
We use the simplest model loaded beam shown in the diagram. The simplest analogy of a beam can be a wooden ruler, photo.

In our case, the beam:

1. It has a rectangular cross-section S=b*h, the length of the supporting part is L;
2. The ruler is loaded with a force Q passing through the center of gravity of the bent plane, as a result of which the ends rotate through a small angle θ, with a deflection relative to the initial horizontal position , equal to f ;
3. The ends of the beam rest hingedly and freely on fixed supports; accordingly, there is no horizontal component of the reaction, and the ends of the ruler can move in any direction.

To determine the deformation of a body under load, use the formula of the elastic modulus, which is determined by the ratio E = R/Δ, where E is a reference value, R is force, Δ is the amount of deformation of the body.

Calculate moments of inertia and forces

For our case, the dependence will look like this: Δ = Q/(S E) . For a load q distributed along the beam, the formula will look like this: Δ = q h/(S E) .

What follows is the most important point. The above Young diagram shows the deflection of a beam or the deformation of a ruler as if it were crushed under a powerful press. In our case, the beam is bent, which means that at the ends of the ruler, relative to the center of gravity, two bending moments are applied with different sign. The loading diagram for such a beam is given below.

To transform Young's dependence for the bending moment, it is necessary to multiply both sides of the equality by the shoulder L. We obtain Δ*L = Q·L/(b·h·E) .

If we imagine that one of the supports is rigidly fixed, and an equivalent balancing moment of forces M max = q*L*2/8 will be applied to the second, respectively, the magnitude of the beam deformation will be expressed by the dependence Δх = M x/((h/3) b (h/2) E). The quantity b h 2 /6 is called the moment of inertia and is designated W. The result is Δx = M x / (W E) the fundamental formula for calculating a beam for bending W = M / E through the moment of inertia and bending moment.

To accurately calculate the deflection, you will need to know the bending moment and moment of inertia. The value of the first can be calculated, but the specific formula for calculating a beam for deflection will depend on the conditions of contact with the supports on which the beam is located and the method of loading, respectively, for a distributed or concentrated load. The bending moment from a distributed load is calculated using the formula Mmax = q*L 2 /8. The given formulas are valid only for a distributed load. For the case when the pressure on the beam is concentrated at a certain point and often does not coincide with the axis of symmetry, the formula for calculating the deflection must be derived using integral calculus.

The moment of inertia can be thought of as the equivalent of a beam's resistance to bending load. The magnitude of the moment of inertia for a simple rectangular beam can be calculated using the simple formula W=b*h 3 /12, where b and h are the cross-sectional dimensions of the beam.

It is clear from the formula that the same ruler or board of rectangular cross-section can have a completely different moment of inertia and deflection value if it is placed on supports in the traditional way or placed on an edge. No wonder almost all elements rafter system roofs are made not from 100x150 timber, but from 50x150 boards.

Real sections building structures can have a variety of profiles, from square, circle to complex I-beam or channel shapes. At the same time, determining the moment of inertia and the amount of deflection manually, “on paper”, for such cases becomes a non-trivial task for a non-professional builder.

Formulas for practical use

In practice, most often the opposite task is faced - to determine the safety factor of floors or walls for a specific case based on a known deflection value. In the construction business, it is very difficult to assess the safety factor using other, non-destructive methods. Often, based on the magnitude of the deflection, it is necessary to perform a calculation, estimate the safety factor of the building and general state load-bearing structures. Moreover, based on the measurements taken, it is determined whether the deformation is acceptable, according to the calculation, or whether the building is in emergency condition.

Advice! In the matter of calculating the limit state of a beam based on the amount of deflection, the requirements of SNiP provide an invaluable service. By setting the deflection limit in a relative value, for example, 1/250, building codes greatly facilitate the determination of the emergency condition of a beam or slab.

For example, if you intend to buy a finished building that has stood for quite a long time on problematic soil, it would be useful to check the condition of the ceiling based on the existing deflection. Knowing everything permissible norm deflection and the length of the beam, one can assess without any calculation how critical the condition of the structure is.

Construction inspection, when assessing deflection and assessing the load-bearing capacity of a floor, takes a more complicated route:

• Initially, the geometry of the slab or beam is measured and the deflection value is recorded;
• Based on the measured parameters, the assortment of the beam is determined, then the formula for the moment of inertia is selected using the reference book;
• The moment of force is determined by the deflection and moment of inertia, after which, knowing the material, you can calculate the actual stresses in a metal, concrete or wooden beam.

The question is why is it so difficult if the deflection can be obtained using the formula for calculation for a simple beam on hinged supports f=5/24*R*L 2 /(E*h) under a distributed force. It is enough to know the span length L, the profile height, design resistance R and modulus of elasticity E for a specific floor material.

Advice! Use in your calculations the existing departmental collections of various design organizations, which contain all the necessary formulas for determining and calculating the maximum loaded state in a condensed form.

Conclusion

Most developers and designers of serious buildings act in a similar way. The program is good, it helps to very quickly calculate the deflection and basic loading parameters of the floor, but it is also important to provide the customer with documentary evidence of the results obtained in the form of specific sequential calculations on paper.

Building a diagram Q.

Let's build a diagram M method characteristic points. We place points on the beam - these are the points of the beginning and end of the beam ( D,A ), concentrated moment ( B ), and also mark the middle of a uniformly distributed load as a characteristic point ( K ) is an additional point for constructing a parabolic curve.

We determine bending moments at points. Rule of signs cm. - .

The moment in IN we will define it as follows. First let's define:

Full stop TO let's take in middle area with a uniformly distributed load.

Building a diagram M . Plot AB – parabolic curve(umbrella rule), area ВD – straight slanted line.

For a beam, determine the support reactions and construct diagrams of bending moments ( M) And shear forces (Q).

1. We designate supports letters A And IN and direct support reactions R A And R B .

Compiling equilibrium equations.

Examination

Write down the values R A And R B on design scheme.

2. Constructing a diagram shear forces method sections. We arrange the sections on characteristic areas(between changes). According to the dimensional thread - 4 sections, 4 sections.

sec. 1-1 move left.

The section passes through the area with evenly distributed load, mark the size z 1 to the left of the section before the start of the section. The length of the section is 2 m. Rule of signs For Q - cm.

We build according to the found value diagramQ.

sec. 2-2 move on the right.

The section again passes through the area with a uniformly distributed load, mark the size z 2 to the right from the section to the beginning of the section. The length of the section is 6 m.

Building a diagram Q.

sec. 3-3 move on the right.

sec. 4-4 move on the right.

We are building diagramQ.

3. Construction diagrams M method characteristic points.

Feature point- a point that is somewhat noticeable on the beam. These are the points A, IN, WITH, D , and also a point TO , wherein Q=0 And bending moment has an extremum. also in middle console we will put an additional point E, since in this area under a uniformly distributed load the diagram M described crooked line, and it is built at least according to 3 points.

So, the points are placed, let's start determining the values ​​​​in them bending moments. Rule of signs - see.

Sites NA, AD – parabolic curve(the “umbrella” rule for mechanical specialties or the “sail rule” for construction specialties), sections DC, SV – straight slanted lines.

Moment at a point D should be determined both left and right from point D . The very moment in these expressions Excluded. At the point D we get two values ​​with difference by the amount m – leap by its size.

Now we need to determine the moment at the point TO (Q=0). However, first we define point position TO , designating the distance from it to the beginning of the section as unknown X .

T. TO belongs second characteristic area, its equation for shear force(see above)

But the shear force incl. TO equal to 0 , A z 2 equals unknown X .

We get the equation:

Now knowing X, let's determine the moment at the point TO on the right side.

Building a diagram M . The construction can be carried out for mechanical specialties, putting aside positive values up from the zero line and using the “umbrella” rule.

For a given design of a cantilever beam, it is necessary to construct diagrams of the transverse force Q and the bending moment M, and perform a design calculation by selecting a circular section.

Material - wood, design resistance of the material R=10MPa, M=14kN m, q=8kN/m

There are two ways to construct diagrams in a cantilever beam with a rigid embedment - the usual way, having previously determined the support reactions, and without determining the support reactions, if you consider the sections, going from the free end of the beam and discarding the left part with the embedment. Let's build diagrams ordinary way.

1. Let's define support reactions.

Evenly distributed load q replace with conditional force Q= q·0.84=6.72 kN

In a rigid embedment there are three support reactions - vertical, horizontal and moment; in our case, the horizontal reaction is 0.

We'll find vertical ground reaction R A And supporting moment M A from equilibrium equations.

In the first two sections on the right there is no shear force. At the beginning of a section with a uniformly distributed load (right) Q=0, in the background - the magnitude of the reaction R A.
3. To construct, we will compose expressions for their determination in sections. Let's construct a diagram of moments on fibers, i.e. down.

(the diagram of individual moments has already been constructed earlier)

We solve equation (1), reduce by EI

Static indetermination revealed, the value of the “extra” reaction has been found. You can start constructing diagrams of Q and M for a statically indeterminate beam... We sketch the given diagram of the beam and indicate the magnitude of the reaction Rb. In this beam, reactions in the embedment can not be determined if you move from the right.

Construction Q plots for a statically indeterminate beam

Let's plot Q.

Construction of diagram M

Let us define M at the extremum point - at the point TO. First, let's determine its position. Let us denote the distance to it as unknown " X" Then

We are building a diagram of M.

Determination of shear stresses in an I-section. Let's consider the section I-beam S x =96.9 cm 3 ; Yх=2030 cm 4 ; Q=200 kN

To determine the shear stress, it is used formula,where Q is the shear force in the section, S x 0 is the static moment of the part of the cross section located on one side of the layer in which the tangential stresses are determined, I x is the moment of inertia of the entire cross section, b is the width of the section in the place where shear stress is determined

Let's calculate maximum shear stress:

Let's calculate the static moment for top shelf:

Now let's calculate shear stress:

We are building shear stress diagram:

Design and verification calculations. For a beam with constructed diagrams of internal forces, select a section in the form of two channels from the condition of strength under normal stresses. Check the strength of the beam using the shear stress strength condition and the energy strength criterion. Given:

Let's show a beam with constructed diagrams Q and M

According to the diagram of bending moments, it is dangerous section C, in which M C = M max = 48.3 kNm.

Normal stress strength condition for this beam has the form σ max =M C /W X ≤σ adm . It is necessary to select a section from two channels.

Let's determine the required calculated value axial moment of resistance of the section:

For a section in the form of two channels, we accept according to two channels No. 20a, moment of inertia of each channel I x =1670cm 4, Then axial moment of resistance of the entire section:

Overvoltage (undervoltage) at dangerous points we calculate using the formula: Then we get undervoltage:

Now let's check the strength of the beam based on strength conditions for tangential stresses. According to shear force diagram dangerous are sections on section BC and section D. As can be seen from the diagram, Q max =48.9 kN.

Strength condition for tangential stresses has the form:

For channel No. 20 a: static moment of area S x 1 = 95.9 cm 3, moment of inertia of the section I x 1 = 1670 cm 4, wall thickness d 1 = 5.2 mm, average flange thickness t 1 = 9.7 mm , channel height h 1 =20 cm, shelf width b 1 =8 cm.

For transverse sections of two channels:

S x = 2S x 1 =2 95.9 = 191.8 cm 3,

I x =2I x 1 =2·1670=3340 cm 4,

b=2d 1 =2·0.52=1.04 cm.

Determining the value maximum shear stress:

τ max =48.9 10 3 191.8 10 −6 /3340 10 −8 1.04 10 −2 =27 MPa.

As seen, τ max<τ adm (27MPa<75МПа).

Hence, the strength condition is satisfied.

We check the strength of the beam according to the energy criterion.

From consideration diagrams Q and M follows that section C is dangerous, in which they operate M C =M max =48.3 kNm and Q C =Q max =48.9 kN.

Let's carry out analysis of the stress state at the points of section C

Let's define normal and shear stresses at several levels (marked on the section diagram)

Level 1-1: y 1-1 =h 1 /2=20/2=10cm.

Normal and tangent voltage:

Main voltage:

Level 2−2: y 2-2 =h 1 /2−t 1 =20/2−0.97=9.03 cm.

Main stresses:

Level 3−3: y 3-3 =h 1 /2−t 1 =20/2−0.97=9.03 cm.

Normal and shear stresses:

Main stresses:

Extreme shear stress:

Level 4−4: y 4-4 =0.

(in the middle the normal stresses are zero, the tangential stresses are maximum, they were found in the strength test using tangential stresses)

Main stresses:

Extreme shear stress:

Level 5−5:

Normal and shear stresses:

Main stresses:

Extreme shear stress:

Level 6−6:

Normal and shear stresses:

Main stresses:

Extreme shear stress:

Level 7−7:

Normal and shear stresses:

Main stresses:

Extreme shear stress:

In accordance with the calculations performed stress diagrams σ, τ, σ 1, σ 3, τ max and τ min are presented in Fig.

Analysis these diagram shows, which is in the section of the beam dangerous points are at level 3-3 (or 5-5), in which:

Using energy criterion of strength, we get

From a comparison of equivalent and permissible stresses it follows that the strength condition is also satisfied

(135.3 MPa<150 МПа).

The continuous beam is loaded in all spans. Construct diagrams Q and M for a continuous beam.

1. Define degree of static indetermination beams according to the formula:

n= Sop -3= 5-3 =2, Where Sop – number of unknown reactions, 3 – number of static equations. To solve this beam it is required two additional equations.

2. Let us denote numbers supports from zero in order ( 0,1,2,3 )

3. Let us denote span numbers from the first in order ( ι 1, ι 2, ι 3)

4. We consider each span as simple beam and build diagrams for each simple beam Q and M. What pertains to simple beam, we will denote with index "0", that which relates to continuous beam, we will denote without this index. Thus, is the shear force and bending moment for a simple beam.

Let's consider beam 1st span

Let's define fictitious reactions for the first span beam using tabular formulas (see table “Fictitious support reactions....»)

Beam 2nd span

Beam 3rd span

5. Compose 3 x moments equation for two points– intermediate supports – support 1 and support 2. This is what they will be two missing equations to solve the problem.

The 3-moment equation in general form:

For point (support) 1 (n=1):

For point (support) 2 (n=2):

We substitute all known quantities, taking into account that the moment at the zero support and at the third support are equal to zero, M 0 =0; M 3 =0

Then we get:

Let's divide the first equation by factor 4 for M 2

Divide the second equation by the factor 20 for M 2

Let's solve this system of equations:

Subtract the second from the first equation and get:

We substitute this value into any of the equations and find M 2

Straight bend. Flat transverse bend 1.1. Construction of diagrams of internal force factors for beams 1.2. Construction of diagrams Q and M using equations 1.3. Construction of Q and M diagrams using characteristic sections (points) 1.4. Strength calculations for direct bending of beams 1.5. Main stresses during bending. Full check of beam strength 1.6. The concept of the center of bending 1.7. Determination of displacements in beams during bending. Concepts of beam deformation and conditions of their rigidity 1.8. Differential equation of the curved axis of a beam 1.9. Direct integration method 1.10. Examples of determining displacements in beams using the direct integration method 1.11. Physical meaning of integration constants 1.12. Method of initial parameters (universal equation of the curved axis of a beam) 1.13. Examples of determining displacements in a beam using the initial parameters method 1.14. Determination of displacements using Mohr's method. Rule A.K. Vereshchagina 1.15. Calculation of the Mohr integral according to the rule of A.K. Vereshchagina 1.16. Examples of determining displacements using the Mohr integral Bibliography 4 1. Direct bending. Flat transverse bend. 1.1. Constructing diagrams of internal force factors for beams Direct bending is a type of deformation in which two internal force factors arise in the cross sections of the rod: a bending moment and a transverse force. In a particular case, the shear force can be zero, then the bending is called pure. In flat transverse bending, all forces are located in one of the main planes of inertia of the rod and perpendicular to its longitudinal axis, and moments are located in the same plane (Fig. 1.1, a, b). Rice. 1.1 The transverse force in an arbitrary cross section of a beam is numerically equal to the algebraic sum of the projections onto the normal to the beam axis of all external forces acting on one side of the section under consideration. The transverse force in the m-n section of the beam (Fig. 1.2, a) is considered positive if the resultant of external forces to the left of the section is directed upward, and to the right - downward, and negative - in the opposite case (Fig. 1.2, b). Rice. 1.2 When calculating the transverse force in a given section, external forces lying to the left of the section are taken with a plus sign if they are directed upwards, and with a minus sign if they are directed downwards. For the right side of the beam - vice versa. 5 The bending moment in an arbitrary cross section of a beam is numerically equal to the algebraic sum of the moments about the central axis z of the section of all external forces acting on one side of the section under consideration. The bending moment in the m-n section of the beam (Fig. 1.3, a) is considered positive if the resultant moment of external forces to the left of the section is directed clockwise, and to the right - counterclockwise, and negative - in the opposite case (Fig. 1.3, b). Rice. 1.3 When calculating the bending moment in a given section, the moments of external forces lying to the left of the section are considered positive if they are directed clockwise. For the right side of the beam - vice versa. It is convenient to determine the sign of the bending moment by the nature of the deformation of the beam. The bending moment is considered positive if, in the section under consideration, the cut-off part of the beam bends convexly downward, i.e., the lower fibers are stretched. In the opposite case, the bending moment in the section is negative. There are differential relationships between the bending moment M, shear force Q and load intensity q. 1. The first derivative of the shear force along the abscissa of the section is equal to the intensity of the distributed load, i.e. . (1.1) 2. The first derivative of the bending moment along the abscissa of the section is equal to the transverse force, i.e. (1.2) 3. The second derivative along the abscissa of the section is equal to the intensity of the distributed load, i.e. (1.3) We consider the distributed load directed upward positive. A number of important conclusions follow from the differential relationships between M, Q, q: 1. If on the beam section: a) the transverse force is positive, then the bending moment increases; b) the shear force is negative, then the bending moment decreases; c) the transverse force is zero, then the bending moment has a constant value (pure bending); 6 d) the transverse force passes through zero, changing sign from plus to minus, max M M, in the opposite case M Mmin. 2. If there is no distributed load on the beam section, then the transverse force is constant, and the bending moment changes according to a linear law. 3. If there is a uniformly distributed load on a section of the beam, then the transverse force changes according to a linear law, and the bending moment - according to the law of a square parabola, convexly facing in the direction of the load (in the case of constructing diagram M from the side of stretched fibers). 4. In the section under a concentrated force, diagram Q has a jump (by the magnitude of the force), diagram M has a kink in the direction of the force. 5. In the section where a concentrated moment is applied, the diagram M has a jump equal to the value of this moment. This is not reflected in the Q diagram. For complex loading of beams, diagrams of transverse forces Q and bending moments M are constructed. Diagram Q(M) is a graph showing the law of change in transverse force (bending moment) along the length of the beam. Based on the analysis of diagrams M and Q, dangerous sections of the beam are determined. Positive ordinates of the Q diagram are laid upward, and negative ordinates are laid down from the base line drawn parallel to the longitudinal axis of the beam. Positive ordinates of the M diagram are laid down, and negative ordinates are laid upward, i.e., the M diagram is constructed from the side of the stretched fibers. The construction of Q and M diagrams for beams should begin with determining the support reactions. For a beam with one clamped end and the other free end, the construction of diagrams Q and M can be started from the free end, without determining the reactions in the embedment. 1.2. The construction of Q and M diagrams using the Beam equations is divided into sections within which the functions for the bending moment and shear force remain constant (do not have discontinuities). The boundaries of the sections are the points of application of concentrated forces, pairs of forces and places of change in the intensity of the distributed load. At each section, an arbitrary section is taken at a distance x from the origin of coordinates, and for this section equations for Q and M are drawn up. Using these equations, diagrams of Q and M are constructed. Example 1.1 Construct diagrams of transverse forces Q and bending moments M for a given beam (Fig. 1.4,a). Solution: 1. Determination of support reactions. We compose equilibrium equations: from which we obtain The reactions of the supports are determined correctly. The beam has four sections Fig. 1.4 loads: CA, AD, DB, BE. 2. Construction of diagram Q. Section CA. In section CA 1, we draw an arbitrary section 1-1 at a distance x1 from the left end of the beam. We define Q as the algebraic sum of all external forces acting to the left of section 1-1: 1 Q 3 0 kN. The minus sign is taken because the force acting to the left of the section is directed downward. The expression for Q does not depend on the variable x1. Diagram Q in this section will be depicted as a straight line parallel to the abscissa axis. Section AD. On the section we draw an arbitrary section 2-2 at a distance x2 from the left end of the beam. We define Q2 as the algebraic sum of all external forces acting to the left of section 2-2: The value of Q is constant over the section (does not depend on the variable x2). The Q plot on the section is a straight line parallel to the abscissa axis. Plot DB. On the site we draw an arbitrary section 3-3 at a distance x3 from the right end of the beam. We define Q3 as the algebraic sum of all external forces acting to the right of section 3-3: . The resulting expression is the equation of an inclined straight line. Section BE. On the site we draw a section 4-4 at a distance x4 from the right end of the beam. We define Q as the algebraic sum of all external forces acting to the right of section 4-4: Here the plus sign is taken because the resultant load to the right of section 4-4 is directed downward. Based on the obtained values, we construct Q diagrams (Fig. 1.4, b). 3. Construction of diagram M. Section CA m1. We define the bending moment in section 1-1 as the algebraic sum of the moments of forces acting to the left of section 1-1. – equation of a straight line. Plot. 3We define the bending moment in section 2-2 as the algebraic sum of the moments of forces acting to the left of section 2-2. – equation of a straight line. Plot. 4We define the bending moment in section 3-3 as the algebraic sum of the moments of forces acting to the right of section 3-3. – equation of a quadratic parabola. 9 We find three values ​​at the ends of the section and at the point with coordinate xk, where since here we have kNm. Plot. 1We define the bending moment in section 4-4 as the algebraic sum of the moments of forces acting to the right of section 4-4. – equation of a quadratic parabola, we find three values ​​of M4: Using the obtained values, we construct a diagram of M (Fig. 1.4, c). In sections CA and AD, the Q diagram is limited by straight lines parallel to the abscissa axis, and in sections DB and BE - by inclined straight lines. In sections C, A and B on the Q diagram there are jumps in the magnitude of the corresponding forces, which serves as a check for the correctness of the Q plot. In sections where Q 0, the moments increase from left to right. In areas where Q 0, the moments decrease. Under the concentrated forces there are kinks in the direction of the action of the forces. Under the concentrated moment there is a jump in the magnitude of the moment. This indicates the correctness of the construction of diagram M. Example 1.2 Construct diagrams Q and M for a beam on two supports loaded with a distributed load, the intensity of which varies according to a linear law (Fig. 1.5, a). Solution Determination of support reactions. The resultant of the distributed load is equal to the area of ​​the triangle, which is a diagram of the load and is applied at the center of gravity of this triangle. We compile the sums of the moments of all forces relative to points A and B: Constructing diagram Q. Let's draw an arbitrary section at a distance x from the left support. The ordinate of the load diagram corresponding to the section is determined from the similarity of triangles. The resultant of that part of the load that is located to the left of the section. The transverse force in the section is equal. The transverse force changes according to the law of a square parabola. Equating the equation of the transverse force to zero, we find the abscissa of the section in which the diagram Q passes through zero: The Q plot is shown in Fig. 1.5, b. The bending moment in an arbitrary section is equal to. The bending moment varies according to the law of a cubic parabola: The bending moment has a maximum value in the section where Q 0, i.e. at Diagram M is shown in Fig. 1.5, c. 1.3. Constructing diagrams of Q and M from characteristic sections (points) Using differential dependencies between M, Q, q and the conclusions arising from them, it is advisable to construct diagrams of Q and M from characteristic sections (without drawing up equations). Using this method, the values ​​of Q and M are calculated in characteristic sections. The characteristic sections are the boundary sections of sections, as well as sections where a given internal force factor has an extreme value. Within the limits between the characteristic sections, the outline 12 of the diagram is established on the basis of the differential dependencies between M, Q, q and the conclusions arising from them. Example 1.3 Construct diagrams Q and M for the beam shown in Fig. 1.6, a. We start constructing the Q and M diagrams from the free end of the beam, while the reactions in the embedment need not be determined. The beam has three loading sections: AB, BC, CD. There is no distributed load in sections AB and BC. Shear forces are constant. The Q diagram is limited to straight lines parallel to the x-axis. Bending moments vary linearly. Diagram M is limited by straight lines inclined to the abscissa axis. There is a uniformly distributed load on section CD. Transverse forces vary according to a linear law, and bending moments - according to the law of a square parabola with convexity in the direction of the distributed load. At the boundary of sections AB and BC, the transverse force changes abruptly. At the boundary of sections BC and CD, the bending moment changes abruptly. 1. Construction of diagram Q. We calculate the values ​​of transverse forces Q in the boundary sections of sections: Based on the calculation results, we construct diagram Q for the beam (Fig. 1, b). From diagram Q it follows that the transverse force in section CD is equal to zero in the section located at a distance qa a q  from the beginning of this section. In this section, the bending moment has its maximum value. 2. Construction of diagram M. We calculate the values ​​of bending moments in the boundary sections of sections: At Kx3, the maximum moment in the section. Based on the calculation results, we construct diagram M (Fig. 5.6, c). Example 1.4 Using a given diagram of bending moments (Fig. 1.7, a) for a beam (Fig. 1.7, b), determine the acting loads and construct diagram Q. The circle indicates the vertex of a square parabola. Solution: Let's determine the loads acting on the beam. Section AC is loaded with a uniformly distributed load, since the diagram M in this section is a square parabola. In the reference section B, a concentrated moment is applied to the beam, acting clockwise, since in diagram M we have a jump upward by the magnitude of the moment. In the NE section, the beam is not loaded, since the M diagram in this section is limited by an inclined straight line. The reaction of support B is determined from the condition that the bending moment in section C is equal to zero, i.e. To determine the intensity of the distributed load, we create an expression for the bending moment in section A as the sum of the moments of forces on the right and equate it to zero. Now we determine the reaction of support A. For this Let's create an expression for bending moments in the section as the sum of the moments of forces on the left, from where Fig. 1.7 Check The design diagram of the beam with a load is shown in Fig. 1.7, c. Starting from the left end of the beam, we calculate the values ​​of transverse forces in the boundary sections of the sections: Diagram Q is shown in Fig. 1.7, d. The considered problem can be solved by drawing up functional dependencies for M, Q in each section. Let's choose the origin of coordinates at the left end of the beam. In the AC section, the diagram M is expressed by a square parabola, the equation of which has the form Constants a, b, c are found from the condition that the parabola passes through three points with known coordinates: Substituting the coordinates of the points into the equation of the parabola, we obtain: The expression for the bending moment will be Differentiating the function M1 , we obtain the dependence for the transverse force. After differentiating the function Q, we obtain an expression for the intensity of the distributed load. In the section NE, the expression for the bending moment is presented in the form of a linear function. To determine the constants a and b, we use the conditions that this straight line passes through two points, the coordinates of which are known. We obtain two equations: from which we have a 10, b  20. The equation for the bending moment in the section NE will be After double differentiation of M2, we will find. Using the found values ​​of M and Q, we construct diagrams of bending moments and shear forces for the beam. In addition to the distributed load, concentrated forces are applied to the beam in three sections, where there are jumps on diagram Q and concentrated moments in the section where there is a shock on diagram M. Example 1.5 For a beam (Fig. 1.8, a), determine the rational position of the hinge C, at which the largest bending moment in the span is equal to the bending moment in the embedment (in absolute value). Construct diagrams of Q and M. Solution Determination of support reactions. Despite the fact that the total number of support links is four, the beam is statically determinate. The bending moment in the hinge C is zero, which allows us to create an additional equation: the sum of the moments about the hinge of all external forces acting on one side of this hinge is equal to zero. Let us compile the sum of the moments of all forces to the right of the hinge C. The diagram Q for the beam is limited by an inclined straight line, since q = const. We determine the values ​​of transverse forces in the boundary sections of the beam: The abscissa xK of the section, where Q = 0, is determined from the equation from which the diagram M for the beam is limited by a square parabola. Expressions for bending moments in sections, where Q = 0, and in the embedment are respectively written as follows: From the condition of equality of moments, we obtain a quadratic equation for the desired parameter x: Real value. We determine the numerical values ​​of transverse forces and bending moments in characteristic sections of the beam. Figure 1.8, b shows the diagram Q, and in Fig. 1.8, c – diagram M. The problem considered could be solved by dividing the hinged beam into its constituent elements, as shown in Fig. 1.8, d. At the beginning, the reactions of the supports VC and VB are determined. Diagrams of Q and M are constructed for the suspended beam SV from the action of the load applied to it. Then they move to the main beam AC, loading it with an additional force VC, which is the pressure force of the beam CB on the beam AC. After that, diagrams Q and M are built for beam AC. 1.4. Strength calculations for direct bending of beams Strength calculations based on normal and shear stresses. When a beam bends directly in its cross sections, normal and tangential stresses arise (Fig. 1.9). Normal stresses are associated with bending moment, shear stresses are associated with shear force. In straight pure bending, the shear stresses are zero. Normal stresses at an arbitrary point in the cross section of a beam are determined by the formula (1.4) where M is the bending moment in a given section; Iz – moment of inertia of the section relative to the neutral axis z; y is the distance from the point where the normal voltage is determined to the neutral z axis. Normal stresses along the height of the section change according to a linear law and reach their greatest value at points farthest from the neutral axis. If the section is symmetrical about the neutral axis (Fig. 1.11), then Fig. 1.11 the greatest tensile and compressive stresses are the same and are determined by the formula - axial moment of resistance of a section during bending. For a rectangular section with width b and height h: (1.7) For a circular section with diameter d: (1.8) For an annular section (1.9) where d0 and d are the inner and outer diameters of the ring, respectively. For beams made of plastic materials, the most rational are symmetrical 20 section shapes (I-beam, box-shaped, annular). For beams made of brittle materials that do not equally resist tension and compression, sections that are asymmetrical with respect to the neutral z-axis (T-beam, U-shaped, asymmetrical I-beam) are rational. For beams of constant cross-section made of plastic materials with symmetrical cross-sectional shapes, the strength condition is written as follows: (1.10) where Mmax is the maximum bending moment in modulus; – permissible stress for the material. For beams of constant cross-section made of plastic materials with asymmetrical cross-sectional shapes, the strength condition is written in the following form: For beams made of brittle materials with sections that are asymmetrical with respect to the neutral axis, if the diagram M is unambiguous (Fig. 1.12), two strength conditions must be written where yP,max , yC,max – distances from the neutral axis to the most distant points of the stretched and compressed zones of the dangerous section, respectively; – permissible stresses in tension and compression, respectively. Fig.1.12. 21 If the diagram of bending moments has sections of different signs (Fig. 1.13), then in addition to checking section 1-1, where Mmax acts, it is necessary to calculate the highest tensile stresses for section 2-2 (with the highest moment of the opposite sign). Rice. 1.13 Along with the main calculation using normal stresses, in a number of cases it is necessary to check the strength of the beam using tangential stresses. Tangential stresses in beams are calculated using the formula of D.I. Zhuravsky (1.13) where Q is the transverse force in the cross section of the beam under consideration; Szотс – static moment relative to the neutral axis of the area of ​​the section part located on one side of a straight line drawn through a given point and parallel to the z axis; b – section width at the level of the point under consideration; Iz is the moment of inertia of the entire section relative to the neutral z axis. In many cases, maximum shear stresses occur at the level of the neutral layer of the beam (rectangle, I-beam, circle). In such cases, the strength condition for tangential stresses is written in the form, (1.14) where Qmax is the largest transverse force in absolute value; – permissible shear stress for the material. For a rectangular section of a beam, the strength condition has the form 22 (1.15) A – cross-sectional area of ​​the beam. For a circular section, the strength condition is presented in the form (1.16) For an I-section, the strength condition is written as follows: (1.17) where Szo,тmсax is the static moment of the half-section relative to the neutral axis; d – wall thickness of the I-beam. Typically, the cross-sectional dimensions of a beam are determined from the strength condition under normal stresses. Checking the strength of beams by shear stress is mandatory for short beams and beams of any length if there are concentrated forces of large magnitude near the supports, as well as for wooden, riveted and welded beams. Example 1.6 Check the strength of a box-section beam (Fig. 1.14) by normal and tangential stresses, if 0 MPa. Construct diagrams in the dangerous section of the beam. Rice. 1.14 Solution 23 1. Constructing diagrams of Q and M using characteristic sections. Considering the left side of the beam, we obtain The diagram of transverse forces is shown in Fig. 1.14, c. . The diagram of bending moments is shown in Fig. 5.14, g. 2. Geometric characteristics of cross section 3. The highest normal stresses in section C, where Mmax acts (modulo): The maximum normal stresses in the beam are almost equal to the permissible ones. 4. The greatest tangential stresses in section C (or A), where the static moment of the half-section area relative to the neutral axis acts; b2 cm – section width at the level of the neutral axis. 5. Tangential stresses at a point (in the wall) in section C: Here is the static moment of the area of ​​the part of the section located above the line passing through point K1; b2 cm – wall thickness at point K1. Diagrams for section C of beam are shown in Fig. 1.15. Example 1.7 For the beam shown in Fig. 1.16, a, required: 1. Construct diagrams of transverse forces and bending moments along characteristic sections (points). 2. Determine the dimensions of the cross section in the form of a circle, rectangle and I-beam from the condition of strength under normal stresses, compare the cross-sectional areas. 3. Check the selected dimensions of beam sections according to tangential stress. Solution: 1. Determine the reactions of the beam supports from where Checking: 2. Constructing diagrams Q and M. Values ​​of transverse forces in characteristic sections of the beam In sections CA and AD, load intensity q = const. Consequently, in these areas the Q diagram is limited to straight lines inclined to the axis. In section DB, the intensity of the distributed load is q = 0, therefore, in this section, the diagram Q is limited to a straight line parallel to the x axis. The Q diagram for the beam is shown in Fig. 1.16, b. Values ​​of bending moments in characteristic sections of the beam: In the second section, we determine the abscissa x2 of the section in which Q = 0: Maximum moment in the second section Diagram M for the beam is shown in Fig. 1.16, c. 2. We create a strength condition based on normal stresses, from which we determine the required axial moment of resistance of the section from the expression determined by the required diameter d of a beam of a circular section. Area of ​​a circular section. For a beam of a rectangular section. Required height of the section. Area of ​​a rectangular section. Determine the required number of the I-beam. Using the tables of GOST 8239-89, we find the nearest higher value of the axial moment of resistance which corresponds to I-beam No. 33 with the characteristics: Tolerance check: (underload by 1% of the permissible 5%) the nearest I-beam No. 30 (W  472 cm3) leads to significant overload ( more than 5%). We finally accept I-beam No. 33. We compare the areas of the round and rectangular sections with the smallest area A of the I-beam: Of the three sections considered, the most economical is the I-beam section. 3. We calculate the highest normal stresses in the dangerous section 27 of the I-beam (Fig. 1.17, a): Normal stresses in the wall near the flange of the I-beam section The diagram of normal stresses in the dangerous section of the beam is shown in Fig. 1.17, b. 5. Determine the highest shear stresses for the selected sections of the beam. a) rectangular section of the beam: b) round section of the beam: c) I-beam section: Tangential stresses in the wall near the flange of the I-beam in dangerous section A (right) (at point 2): The diagram of tangential stresses in dangerous sections of the I-beam is shown in Fig. 1.17, c. The maximum tangential stresses in the beam do not exceed the permissible stresses. Example 1.8 Determine the permissible load on the beam (Fig. 1.18, a) if the cross-sectional dimensions are given (Fig. 1.19, a). Construct a diagram of normal stresses in a dangerous section of a beam at an allowable load. Figure 1.18 1. Determination of reactions of beam supports. Due to the symmetry of the system VVB A8qa . 29 2. Construction of Q and M diagrams using characteristic sections. Transverse forces in characteristic sections of a beam: Diagram Q for a beam is shown in Fig. 5.18, b. Bending moments in characteristic sections of the beam For the second half of the beam, the ordinates M are along the axes of symmetry. Diagram M for the beam is shown in Fig. 1.18, b. 3. Geometric characteristics of the section (Fig. 1.19). We divide the figure into two simple elements: I-beam - 1 and rectangle - 2. Fig. 1.19 According to the assortment for I-beam No. 20, we have For a rectangle: Static moment of the sectional area relative to the z1 axis Distance from the z1 axis to the center of gravity of the section Moment of inertia of the section relative to the main central axis z of the entire section according to the formulas for the transition to parallel axes 4. Strength condition for normal stresses for dangerous point “a” (Fig. 1.19) in dangerous section I (Fig. 1.18): After substituting numerical data 5. With an allowable load q in a dangerous section, the normal stresses at points “a” and “b” will be equal: Diagram normal stresses for dangerous section 1-1 is shown in Fig. 1.19, b. Example 1.9 Determine the required dimensions of the cross-section of a cast-iron beam (Fig. 1.20), having previously selected a rational location of the section. Make Decision 1. Determine the reactions of the beam supports. 2. Construction of Q and M diagrams. The diagrams are presented in Fig. 1.20, in, g. The largest (in absolute value) bending moment occurs in section “b”. In this section, the stretched fibers are located at the top. Most of the material should be located in the tension zone. Therefore, it is rational to position the beam section as shown in Fig. 1.20, b. 3. Determination of the position of the center of gravity of the section (by analogy with the previous example): 4. Determination of the moment of inertia of the section relative to the neutral axis: 5. Determination of the required dimensions of the beam section from the condition of strength under normal stresses. Let us denote by y, respectively, the distances from the neutral axis to the most distant points in the tension and compression zones (for section B): then the points of the tension zone that are most distant from the neutral axis are dangerous. We create a strength condition for point m in section B: or after substituting numerical values. In this case, the stresses at point n, the most distant from the neutral axis in the compressed zone (in section B), will be MPa. Diagram M is ambiguous. It is necessary to check the strength of the beam in section C. Here is the moment, but the lower fibers are stretched. The dangerous point will be point n: In this case, the stresses at point m will be From the calculations we finally accept The diagram of normal stresses for the dangerous section C is shown in Fig. 1.21. Rice. 1.21 1.5. Main stresses during bending. Full check of the strength of beams Above, examples of calculating beams for strength using normal and shear stresses are discussed. In the vast majority of cases, this calculation is sufficient. However, in thin-walled beams of I-beam, T-beam, channel and box sections, significant shear stresses arise at the junction of the wall and the flange. This occurs in cases where a significant shear force is applied to the beam and there are sections in which M and Q are simultaneously large. One of these sections will be dangerous and is checked 34 by principal stresses using one of the strength theories. Checking the strength of beams using normal, tangential and principal stresses is called a complete check of the strength of beams. This calculation is discussed below. The main thing is to calculate the beam using normal stresses. The strength condition for beams, the material of which equally resists tension and compression, has the form where Mmax ─ maximum bending moment (modulo), taken from the diagram M, Wz ─ axial moment of resistance of the section relative to the neutral axis of the beam; [ ]─ permissible normal stress for the material. From the strength condition (1), the required cross-sectional dimensions of the beam are determined. The selected dimensions of the beam section are checked by shear stresses. The strength condition for tangential stresses has the form (formula of D.I. Zhuravsky): where Qmax ─ maximum transverse force taken from the diagram Q; Szots.─ static moment (relative to the neutral axis) of the cut-off part of the cross section located on one side of the level at which shear stresses are determined; I z ─ moment of inertia of the entire cross section relative to the neutral axis; b─ the width of the beam section at the level where the shear stresses are determined; ─ permissible tangential stress of the material during bending. The normal stress strength test refers to the point furthest from the neutral axis in the section where Mmax acts. The shear stress strength test refers to a point located on the neutral axis in the section where Qmax acts. In beams with a thin-walled cross-section (I-beam, etc.), a point located in the wall in a section where M and Q are both large can be dangerous. In this case, the strength is checked using principal stresses. The main and extreme tangential stresses are determined by analytical dependencies obtained from the theory of the plane stress state of bodies: The angle of inclination of the main areas is determined by the formula (1.22) Having the values ​​of the main stresses, strength conditions are drawn up according to one or another strength theory. For example, According to the third theory of the highest tangential stresses, we have After substituting the values ​​of the principal stresses, we finally obtain (1.23) According to the fourth energy theory of strength, the strength condition has the form (1.24) From formulas (1.6) and (1.7) it is clear that the design stress Eq depends on. Consequently, the material element of the beam for which it will be large at the same time is subject to verification. This is carried out in the following cases: 1) the bending moment and shear force reach their greatest value in the same section; 2) the width of the beam changes sharply near the edges of the section (I-beam, etc.). If the specified conditions do not hold, then it is necessary to consider several sections in which the highest values ​​of eq. Example 1.10 A welded beam of I-beam cross-section with a span of l = 5 m, simply supported at the ends, is loaded with a uniformly distributed load of intensity q and a concentrated force P 5qa applied at a distance a = 1 m from the right support (Fig. 1.22). Determine the permissible load on the beam from the strength condition for normal stresses and check for tangential and principal stresses according to 36 4th (energy) strength theory. Construct diagrams in a dangerous section using principal stresses and examine the stressed state of an element selected in the wall near the flange in the indicated section. Allowable tensile and compressive stress: bending 160 MPa; and shear 100 MPa. Rice. 1.22 Solution 1. Determination of reactions of beam supports: 2. Construction of diagrams M and Q using characteristic sections (points): 3. Calculation of geometric characteristics of the beam section. a) axial moment of inertia of the section relative to the neutral z-axis: 37 b) Axial moment of resistance relative to the neutral z-axis: 4. Determination of the permissible load on the beam from the condition of strength by normal stresses: Permissible load on the beam 5. Checking the strength of the beam by tangential stresses using the formula D.I. Zhuravsky Static moment of the half-section of an I-beam relative to the neutral axis z: Section width at point level 3: Maximum transverse force Maximum shear stresses in the beam 6. Checking the strength of the beam by principal stresses. Dangerous in terms of principal stresses is the section D, in which M and Q are both large, and the dangerous points in this section are points 2 and 4, where  and  are both large (Fig. 1.23). For points 2 and 4, we check the strength by principal stresses, using the 4th theory of strength where (2) and (2)─ normal and shear stresses at point 2(4), respectively (Fig. 1.2). Rice. 1.23 distance from the neutral axis to point 2. where Sz is the static moment of the flange relative to the neutral axis z. cm ─ section width along a line passing through point 3. Equivalent stresses according to the 4th theory of strength at point 2 of section D: The strength condition according to the 4th theory of strength is satisfied. 7. Construction of diagrams of normal, tangential, principal and extreme tangential stresses in dangerous section D (based on principal stresses). a) calculate the stresses at points (1-5) of section D using the appropriate formulas. Point 2 (in the wall) Previously, the values ​​of normal and shear stresses at point 2 were calculated. We find the main and extreme shear stresses at the same point 2: Point 3. Normal and shear stresses at point 3: Main and extreme shear stresses at point 3: The voltages at points 4 and 5 are found similarly. Based on the data obtained, we construct diagrams, max. 8. The stressed state of the element selected in the vicinity of point 2 in section D is shown in Fig. 1.24, angle of inclination of the main platforms 1.6. The concept of the center of bending As mentioned above, the tangential stresses in the cross sections of thin-walled rods during bending (for example, an I-beam or a channel) are determined by the formula In Fig. 194 shows diagrams of tangential stresses in an I-section. Using the technique described in paragraph 63, you can construct diagram 41 also for the channel. Let's consider the case when the channel is embedded in a wall, and at the other end it is loaded with a force P applied at the center of gravity of the section. Rice. 1.25 The general view of the τ diagram in any section is shown in Fig. 1.25, a. Tangential stresses τу arise in the vertical wall. As a result of the action of stresses τу, a total shear force T2 arises (Fig. 1.25, b). If we neglect the tangential stresses τу in the flanges, then we can write the approximate equality In horizontal flanges, tangential stresses τх arise, which are directed horizontally. The greatest shear stress in the flange τx max is equal to Here S1OTS is the static moment of the flange area relative to the Ox axis: Consequently, the Total shear force in the flange will be determined as the area of ​​the shear stress diagram multiplied by the thickness of the flange. Exactly the same shear force acts on the lower flange as on top, but it is directed in the opposite direction. Two forces T1 form a pair with a moment (1.25) Thus, due to the tangential stresses τу and τх, three internal tangential forces arise, which are shown in Fig. 1.25, b. From this figure it is clear that the forces T1 and T2 tend to rotate the channel section relative to the center of gravity in the same direction. Rice. 1.25 Consequently, an internal torque appears in the channel section, directed clockwise. So, when a channel beam is bent by a force applied at the center of gravity of the section, the beam simultaneously twists. Three tangential forces can be reduced to a principal vector and a principal moment. The magnitude of the main moment depends on the position of the point to which the forces are brought. It turns out that it is possible to choose a point A relative to which the main moment is equal to zero. This point is called the center of the bend. Equating the moment of tangential forces to zero: we obtain Taking into account expression (1.25), we will finally find the distance from the axis of the vertical wall to the center of bending: If an external force is applied not at the center of gravity of the section, but at the center of bending, then it will create the same moment relative to the center of gravity as create internal tangential forces, but only of the opposite sign. With such a load (Fig. 1.25, c), the channel will not twist, but will only bend. That is why point A is called the center of the bend. A detailed description of the calculation of thin-walled rods is given in Chapter. XIII. 1.7. Determination of displacements in beams during bending. Concepts of deformation of beams and conditions for their rigidity Under the influence of an external load, the beam is deformed and its axis is bent. The curve into which the axis of the beam turns after applying a load is called an elastic line, provided that the stresses of the beam do not exceed the proportionality limit. Depending on the direction of the load, the location of the diagrams, the elastic line can have a convexity upward (Fig. 1.26, a), downward (Fig. 1.26, b) or a combination (Fig. 1.26, c). In this case, the centers of gravity of the cross sections move respectively either up or down, and the sections themselves rotate relative to the neutral axis, remaining perpendicular to the curved axis of the beam (Fig. 1.26, a). Strictly speaking, the centers of gravity of the cross sections also move in the direction of the longitudinal axis of the beam. However, due to the smallness of these movements for beams, they are neglected, i.e., it is assumed that the center of gravity of the section moves perpendicular to the axis of the beam. Let us denote this movement by y, and in the future we will understand by it the deflection of the beam (see Fig. 1.26). The deflection of a beam in a given section is the movement of the center of gravity of the section in a direction perpendicular to the axis of the beam. Rice. 1.26 Deflections in various sections of a beam depend on the position of the sections and are a variable value. So, for a beam (Fig. 1.26, a) at point B the deflection will have a maximum value, and at point D it will be zero. As already noted, along with the movement of the center of gravity of the section, the sections rotate relative to the neutral axis of the section. The angle by which the section is rotated relative to its original position is called the angle of rotation of the section. We will denote the angle of rotation by (Fig. 1.26, a). Since when a beam is bent, the cross section always remains perpendicular to its curved axis, the angle of rotation can be represented as the angle between the tangent to the curved axis at a given point and the original axis of the beam (Fig. 1.26, a) or perpendicular to the original and curved axes of the beam at the point in question. The angle of rotation of the section for beams is also a variable value. For example, for a beam (Fig. 1.26, b), it has a maximum value in the hinged supports, and a minimum value of 0 for the section in which the deflection has a maximum value. For a cantilever beam (Fig. 1.26, a) the maximum angle of rotation will be at its free end, i.e. at point B. To ensure normal operation of beams, it is not enough that they satisfy the strength condition. It is also necessary that the beams have sufficient rigidity, that is, that the maximum deflection and angle of rotation do not exceed the permissible values ​​determined by the operating conditions of the beams. This situation is called the condition of beam rigidity during bending. In a short mathematical form of notation, the stiffness conditions have the form: where [y] and, accordingly, the permissible deflection and angle of rotation. 45 The permissible deflection is usually specified as part of the distance between the supports of the beam (span length l), i.e. where m is a coefficient depending on the value and operating conditions of the system in which this beam is used. In each branch of mechanical engineering, this value is determined by design standards and varies widely. As follows: - for crane beams m = 400 - 700; - for railway bridges m = 1000; - for spindles of lathes m= 1000-2000. The permissible angles of rotation for beams usually do not exceed 0.001 rad. The left side of equations (1.26) includes the maximum deflection ymax and rotation angle max, which are determined by calculation based on known methods: analytical, graphical and graphic-analytical, some of which are discussed below. 1.8. Differential equation for the curved axis of a beam Under the influence of external forces, the axis of the beam is bent (see Fig. 1.26, a). Then the equation of the curved axis of the beam can be written in the form and the angle of rotation  for any section will be equal to the angle of inclination of the tangent to the curved axis at a given point. The tangent of this angle is numerically equal to the derivative of the deflection along the abscissa of the current section x, i.e. Since the deflections of the beam are small compared to its length l (see above), we can assume that the angle of rotation (1.27) When deriving the normal stress formula during bending, it was found that the following relationship exists between the curvature of the neutral layer and the bending moment: This formula shows that the curvature changes along the length of the beam according to the same law according to which the value Mz changes. If a beam of constant cross-section experiences pure bending (Fig. 5.27), in which the moment along the length does not change, its curvature is: Therefore, for such a beam, the radius of curvature is also a constant value and the beam in this case will bend along a circular arc. However, in the general case, it is not possible to directly apply the law of change in curvature to determine deflections. To solve the problem analytically, we use the well-known expression for curvature from mathematics. (1.29) Substituting (1.28) into (1.29), we obtain the exact differential equation for the curved axis of the beam: . (1.30) Equation (1.30) is nonlinear, and its integration is associated with great difficulties. Considering that deflections and rotation angles for real beams used in mechanical engineering, construction, etc. are small, then the value can be neglected. Taking into account this, and also the fact that for the right coordinate system the bending moment and curvature have the same sign (Fig. 1.26), then for the right coordinate system the minus sign in equation (1.26) can be omitted. Then the approximate differential equation will have the form 1.9. Direct integration method This method is based on the integration of equation (1.31) and allows us to obtain the equation of the elastic axis of the beam in the form of deflections y f (x) and the equation of rotation angles. Having integrated equation (1.31) for the first time, we obtain the equation of rotation angles (1.32) where C is the integration constant . Integrating a second time, we obtain the deflection equation where D is the second constant of integration. The constants C and D are determined from the boundary conditions of the support of the beam and the boundary conditions of its sections. So for a beam (Fig. 1.26, a), at the place of embedding (x l), the deflection and angle of rotation of the section are equal to zero, and for a beam (see Fig. 1.26, b) the deflection y and deflection yD 0, at x .l For hinged supported beam with consoles (Fig. 1.28), when the origin of coordinates is aligned with the end of the left support and the choice of the right coordinate system, the boundary conditions have the form Taking into account the boundary conditions, the integration constants are determined. After substituting the integration constants into the equations of rotation angles (1.32) and deflections (1.33), the rotation angles and deflections of a given section are calculated. 1.10. Examples of determining displacements in beams using the direct integration method Example 1.11 Determine the maximum deflection and angle of rotation for a cantilever beam (Fig. 1.26, a). Solution The origin of coordinates is aligned with the left end of the beam. The bending moment in an arbitrary section at a distance x from the left end of the beam is calculated using the formula Taking into account the moment, the approximate differential equation has the form Integrating for the first time, we have (1.34) Integrating for the second time Boundary conditions Taking into account the second condition, from which Similarly, from the first condition we will have Taking into account of the found integration constants C and D, the equation for the angles of rotation and deflection will have the form: When (see Fig. 1.26, a) the angle of rotation and deflection have maximum values: A positive value of the angle  indicates that the section when bending the beam rotates in the direction opposite to the movement clockwise. A negative y value indicates that the center of gravity of the section is moving downward. 1.11. Physical meaning of the integration constants If we turn to equations (1.32), (1.33) and (1.34), (1.35), the examples considered above, then it is easy to notice that for x 0 it follows from them. Thus, we can conclude that the integration constants C and D represent the product of the beam stiffness, respectively, by the angle: rotation 0 and deflection y0 at the origin. Dependencies (1.36) and (1.37) always turn out to be valid for beams that have one loading section, if we calculate the bending moment from the forces located between the section and the origin. The same remains valid for beams with any number of loading sections, if special techniques for integrating the differential equation of the curved axis of the beam are used, which will be discussed below. 1.12. Method of initial parameters (universal equation of the curved axis of a beam) When determining deflections and rotation angles by the method of direct integration, it is necessary to find two integration constants C and D, even in cases where the beam has one loading section. In practice, beams are used that have several loading areas. In these cases, the bending moment law will be different in different loading areas. Then the differential equation of the curved axis will need to be compiled for each section of the beam and for each of them its constants of integration C and D must be found. Obviously, if a beam has n loading sections, then the number of integration constants will be equal to twice the number of sections. To determine them, you will need to solve 2 equations. This task is time consuming. To solve problems that have more than one loading area, the method of initial parameters, which is a development of the method of direct integration, has become widespread. It turns out that by observing certain conditions and techniques for composing and integrating equations over sections, it is possible to reduce the number of integration constants, regardless of the number of loading sections, to two, representing the deflection and rotation angle at the origin. Let us consider the essence of this method using the example of a cantilever beam (Fig. 1.28), loaded with an arbitrary load, but creating a positive moment in any section of the beam. Let a beam of constant cross-section be given, and the cross-section has an axis of symmetry coinciding with the y-axis, and the entire load is located in one plane passing through this axis. Let us set the task of establishing the dependencies that determine the angle of rotation and deflection of an arbitrary section of a beam. Rice. 1.29 When solving problems, we agree: 1. The origin of coordinates will be associated with the left end of the beam, and it is common to all sections. 2. The bending moment in an arbitrary section will always be calculated for the section of the beam located to the left of the section, i.e., between the origin and the section. 3. We will integrate the differential equation of the curved axis in all sections without opening the brackets of some expressions containing brackets. So, for example, integration of an expression of the form P x(b) is carried out without opening the parentheses, namely according to the following formula. Integration according to this formula differs from integration with preliminary opening of the brackets only in the value of an arbitrary constant. 4. When composing an expression for the bending moment in an arbitrary section caused by an external concentrated moment M, we will add the factor (x)a0 1. Adhering to these rules, we will compose and integrate an approximate differential equation for each of the five sections of the beam indicated in Fig. 1.28 in Roman numerals. The approximate differential equation for the indicated sections has the same form: (1.38) but for each section the bending moment has its own law of change. The bending moments for the sections have the form: Substituting the expressions for the bending moment into equation (1.38), for each of the sections after integration we obtain two equations: the equation of rotation angles and the equation of deflections, which will include their two integration constants Ci and Di. Since the beam has five sections, there will be ten such integration constants. However, taking into account that the curved axis of the beam is a continuous and elastic line, then at the boundaries of adjacent sections the deflection and the angle of rotation have the same values, i.e., etc. Because of this, from a comparison of the equations for the angles of rotation and deflections of neighboring sections, we obtain that the constants of integration Thus, instead of ten constants of integration, to solve the problem posed, it is necessary to determine only two constants of integration C and D. From the consideration of the integral equations of the first section it follows that at x 0: i.e. they represent the same dependencies (1.36) and (1.37). The initial parameters 0 and y0 о are determined from the boundary conditions that were discussed in the previous section. Analyzing the obtained expressions for the angles of rotation and deflections y, we see that the most general form of the equations corresponds to the fifth section. Taking into account the integration constants, these equations have the form: The first of these equations represents the equation of rotation angles, and the second represents the equation of deflections. Since more than one concentrated force can act on a beam, a moment or a beam can have more than one section with a distributed load, then for the general case, equations (1.38), (1.39) will be written in the form: Equations (1.41), (1.42) are called universal equations the curved axis of the beam. The first of these equations is the equation of rotation angles, and the second is the equation of deflections. Using these equations, it is possible to determine the deflections and angles of rotation of sections for any statically determinate beams whose stiffness along their length is constant EI  const. In equations (1.41), (1.42): M, P, q, qx ─ external load located between the origin of coordinates and the section in which the displacements (angle of rotation and deflection) are determined; a, b, c, d ─ distances from the origin of coordinates to the points of application, respectively, of the moment M, concentrated force P, the beginning of a uniformly distributed load and the beginning of an unevenly distributed load. It is necessary to pay attention: 53 1. In the opposite direction of the external load, which is accepted when deriving universal equations, in front of the corresponding term of the equations the sign changes to the opposite, i.e., to minus. 2. The last two terms of equations (1.41), (1.42) are valid only if the distributed load does not terminate before the section in which the deflection and angle of rotation are determined. If the load does not reach this section, then it must be continued to this section and at the same time added on the extended section the same distributed load, but of opposite sign, this idea is explained in Fig. 1.30. The dotted line shows the added distributed load on the extended section. Rice. 1.30 When determining rotation angles  and deflections y, the origin of coordinates should be placed at the left end of the beam, directing the y axis upward and the x axis to the right. The compiled equation for rotation angles and deflections includes only those forces that are located to the left of the section, i.e. on the section of the beam between the origin of coordinates and the section in which the deflection and angle of rotation are determined (including the forces acting in the section coinciding with the origin of coordinates). 1.13. Examples of determining displacements in a beam using the initial parameters method Example 1.12 For a beam (Fig. 1.31), clamped at the left end and loaded with a concentrated force P, determine the angle of rotation and deflection at the point of application of the force, as well as the free end (section D). Beam stiffness Fig. 1.31 Solution of the static equilibrium equation: 1) Note that the reactive torque is directed counterclockwise, so it will enter the equation of the curved axis with a minus sign. 2. Combine the origin of coordinates with point B and set the initial parameters. In pinching ()B there is no deflection and no rotation angle, i.e. 0 0. We write down the equation of rotation angles and deflections for an arbitrary section of the second section, i.e. located at a distance x from the origin of coordinates Taking into account the reactive forces, as well as the equality to zero of the initial parameters, these equations have the form For x l we have the angle of rotation and deflection of the section C, respectively 55 For section D, x1l 12(1)2 Example 1.13 Determine the maximum deflection and angle rotation on the right support of the beam, loaded in the middle of the span with a concentrated force (Fig. 1.32). Solution 1. Determine the support reactions From the static equations we have B 2. Place the origin of coordinates at the left end of the beam (point B). Rice. 1.32 3. Set the initial parameters. Deflection at the origin By0, since the support does not allow vertical movement. It should be noted that if the support were spring-loaded, then the deflection at the origin would be equal to the deformation of the spring. The angle of rotation at the origin of coordinates is not equal to zero, i.e. 4. Determine the angle of rotation at the origin of coordinates 0. To do this, we use the condition that at x l the deflection is equal to zero yD 0: 3 Since the beam is symmetrical relative to the load P, the angle of rotation on the right support is equal to the angle of rotation on the left support. 2 BD 16z Pl EI . The maximum deflection will be in the middle of the beam at x. Therefore, Example 1.14 Determine the deflection in the middle of the span and at the right end of the beam (Fig. 1.33), if the beam is made of I-beam No. 10 (moment of inertia Iz 198 scm4), loaded with a distributed load q 2.N/m, concentrated by a moment M force. P kkNN Fig. 1.33 Solution 1. Determining the support reactions From where: Checking the correctness of determining the reactions 2. Combine the origin of coordinates with point B and set the initial parameters. From Fig. 1.33 it follows that at the origin of coordinates the deflection y0 0 and the angle of rotation. 57 3. Determine the initial parameters y0 and 0. To do this, we use the boundary conditions that when: To implement the boundary conditions, we create an equation for the curved axis. for two sections: section BC 0 mm1: When writing this equation, it was taken into account that the distributed load was interrupted at point C, therefore, according to what was said above, it was continued and a compensating load of the same magnitude, but in the opposite direction, was introduced in the continued section. Taking into account the boundary conditions (point 3) and the load, equations (1.43) and (1.44) have the form: From the joint solution of these equations we have 4. We determine the deflection in sections K and E. For section K at x 2 mm we have 1.14. Determination of displacements using Mohr's method Rule A.K. Vereshchagin's Mohr method is a general method for determining displacements in linearly deformable rod systems. The determination of displacements (linear, angular) in the design sections is made using the Mohr formula (integral), which is easy to obtain based on the theorem on the reciprocity of work (Betti’s theorem) and the theorem on the reciprocity of displacements (Maxwell’s theorem). Let, for example, be given a flat elastic system in the form of a beam (Fig. 1.34), loaded with a flat balanced arbitrary load. We will call the given state of the system cargo and denote it by the letter P. Under the influence of an external load, deformation will occur, and displacements will occur at point K, in particular, in the direction perpendicular to the axis - deflection cr. Let us introduce a new (auxiliary) state of the same system, but loaded at point K in the direction of the desired displacement (cr) by a unit dimensionless force (Fig. 1.34). We will denote such a state of the system by the letter i, and will call it a single state. 59 Fig. 1.34 Based on Betti’s theorem, the possible work of the forces of the cargo state pi A and the forces of a single state pi A are equal to (1.45) The possible work of the forces of the cargo state, expressed in terms of internal forces, is determined by the formula and the forces of a single state - by the formula (1.47) Taking into account (1.46 ), (1.47) from (1.45) we have (1.48) where M p , Qp, Np ─ respectively the bending moment, transverse and longitudinal forces arising in the system from the external load; Mi, Qi, Ni ─ respectively, bending moment, transverse and longitudinal forces arising in the system from a unit load applied in the direction of the determined displacement; k ─ coefficient taking into account the unevenness of tangential stresses across the section; I ─ axial moment of inertia relative to the main central axis; A─ cross-sectional area of ​​the rod in the area; 60 E, G ─ elastic moduli of the material. The uneven distribution of tangential stresses in a section depends on the shape of the section. For rectangular and triangular sections k 1.2, circular section k 1.11, circular annular section k 2. Formula (1.48) allows you to determine the displacement at any point of a flat elastic system. When determining the deflection in the section (K), we apply a unit force (dimensionless) at this point. In the case of determining the angle of rotation of the section at point K, it is necessary to apply a unit dimensionless moment

We will start with the simplest case, the so-called pure bend.

Pure bending is a special case of bending in which the transverse force in the sections of the beam is zero. Pure bending can only occur when the self-weight of the beam is so small that its influence can be neglected. For beams on two supports, examples of loads causing pure

bending, shown in Fig. 88. In sections of these beams, where Q = 0 and, therefore, M = const; pure bending takes place.

The forces in any section of the beam during pure bending are reduced to a pair of forces, the plane of action of which passes through the axis of the beam, and the moment is constant.

Voltages can be determined based on the following considerations.

1. The tangential components of forces along elementary areas in the cross section of a beam cannot be reduced to a pair of forces, the plane of action of which is perpendicular to the section plane. It follows that the bending force in the section is the result of action along elementary areas

only normal forces, and therefore with pure bending the stresses are reduced only to normal.

2. In order for efforts on elementary sites to be reduced to only a couple of forces, among them there must be both positive and negative. Therefore, both tension and compression fibers of the beam must exist.

3. Due to the fact that the forces in different sections are the same, the stresses at the corresponding points of the sections are the same.

Let's consider some element near the surface (Fig. 89, a). Since no forces are applied along its lower edge, which coincides with the surface of the beam, there are no stresses on it. Therefore, there are no stresses on the upper edge of the element, since otherwise the element would not be in equilibrium. Considering the element adjacent to it in height (Fig. 89, b), we arrive at

The same conclusion, etc. It follows that there are no stresses along the horizontal edges of any element. Considering the elements that make up the horizontal layer, starting with the element near the surface of the beam (Fig. 90), we come to the conclusion that there are no stresses along the lateral vertical edges of any element. Thus, the stress state of any element (Fig. 91, a), and in the limit, fibers, should be represented as shown in Fig. 91,b, i.e. it can be either axial tension or axial compression.

4. Due to the symmetry of the application of external forces, the section along the middle of the length of the beam after deformation should remain flat and normal to the axis of the beam (Fig. 92, a). For the same reason, sections in quarters of the length of the beam also remain flat and normal to the axis of the beam (Fig. 92, b), unless the extreme sections of the beam during deformation remain flat and normal to the axis of the beam. A similar conclusion is valid for sections in eighths of the length of the beam (Fig. 92, c), etc. Consequently, if during bending the outer sections of the beam remain flat, then for any section it remains

It is a fair statement that after deformation it remains flat and normal to the axis of the curved beam. But in this case, it is obvious that the change in elongation of the fibers of the beam along its height should occur not only continuously, but also monotonically. If we call a layer a set of fibers that have the same elongations, then it follows from what has been said that the stretched and compressed fibers of the beam should be located on opposite sides of the layer in which the elongations of the fibers are equal to zero. We will call fibers whose elongations are zero neutral; a layer consisting of neutral fibers is a neutral layer; the line of intersection of the neutral layer with the cross-sectional plane of the beam - the neutral line of this section. Then, based on the previous reasoning, it can be argued that with pure bending of a beam, in each section there is a neutral line that divides this section into two parts (zones): a zone of stretched fibers (stretched zone) and a zone of compressed fibers (compressed zone). ). Accordingly, at the points of the stretched zone of the section, normal tensile stresses should act, at the points of the compressed zone - compressive stresses, and at the points of the neutral line the stresses are equal to zero.

Thus, with pure bending of a beam of constant cross-section:

1) only normal stresses act in sections;

2) the entire section can be divided into two parts (zones) - stretched and compressed; the boundary of the zones is the neutral section line, at the points of which the normal stresses are equal to zero;

3) any longitudinal element of the beam (in the limit, any fiber) is subjected to axial tension or compression, so that adjacent fibers do not interact with each other;

4) if the extreme sections of the beam during deformation remain flat and normal to the axis, then all its cross sections remain flat and normal to the axis of the curved beam.

Stress state of a beam under pure bending

Let us consider an element of a beam subject to pure bending, concluding located between sections m-m and n-n, which are spaced one from the other at an infinitesimal distance dx (Fig. 93). Due to position (4) of the previous paragraph, sections m- m and n - n, which were parallel before deformation, after bending, remaining flat, will form an angle dQ and intersect along a straight line passing through point C, which is the center of curvature neutral fiber NN. Then the part AB of the fiber enclosed between them, located at a distance z from the neutral fiber (the positive direction of the z axis is taken towards the convexity of the beam during bending), will turn after deformation into an arc AB. A piece of neutral fiber O1O2, having turned into an arc, O1O2 will not change its length, while fiber AB will receive an elongation:

before deformation

after deformation

where p is the radius of curvature of the neutral fiber.

Therefore, the absolute lengthening of segment AB is equal to

and relative elongation

Since, according to position (3), fiber AB is subjected to axial tension, then during elastic deformation

This shows that normal stresses along the height of the beam are distributed according to a linear law (Fig. 94). Since the equal force of all forces over all elementary cross-sectional areas must be equal to zero, then

from where, substituting the value from (5.8), we find

But the last integral is a static moment about the Oy axis, perpendicular to the plane of action of the bending forces.

Due to its equality to zero, this axis must pass through the center of gravity O of the section. Thus, the neutral line of the section of the beam is a straight line y, perpendicular to the plane of action of bending forces. It is called the neutral axis of the beam section. Then from (5.8) it follows that the stresses at points lying at the same distance from the neutral axis are the same.

The case of pure bending, in which the bending forces act in only one plane, causing bending only in that plane, is planar pure bending. If the said plane passes through the Oz axis, then the moment of elementary forces relative to this axis should be equal to zero, i.e.

Substituting here the value of σ from (5.8), we find

The integral on the left side of this equality, as is known, is the centrifugal moment of inertia of the section relative to the y and z axes, so

The axes about which the centrifugal moment of inertia of the section is zero are called the main axes of inertia of this section. If they, in addition, pass through the center of gravity of the section, then they can be called the main central axes of inertia of the section. Thus, with flat pure bending, the direction of the plane of action of bending forces and the neutral axis of the section are the main central axes of inertia of the latter. In other words, to obtain a flat, pure bend of a beam, a load cannot be applied to it arbitrarily: it must be reduced to forces acting in a plane that passes through one of the main central axes of inertia of the sections of the beam; in this case, the other main central axis of inertia will be the neutral axis of the section.

As is known, in the case of a section that is symmetrical about any axis, the axis of symmetry is one of its main central axes of inertia. Consequently, in this particular case we will certainly obtain pure bending by applying appropriate loads in a plane passing through the longitudinal axis of the beam and the axis of symmetry of its section. A straight line perpendicular to the axis of symmetry and passing through the center of gravity of the section is the neutral axis of this section.

Having established the position of the neutral axis, it is not difficult to find the magnitude of the stress at any point in the section. In fact, since the sum of the moments of elementary forces relative to the neutral axis yy must be equal to the bending moment, then

whence, substituting the value of σ from (5.8), we find

Since the integral is. moment of inertia of the section relative to the yy axis, then

and from expression (5.8) we obtain

The product EI Y is called the bending stiffness of the beam.

The greatest tensile and largest compressive stresses in absolute value act at the points of the section for which the absolute value of z is greatest, i.e., at the points furthest from the neutral axis. With the notation, Fig. 95 we have

The value Jy/h1 is called the moment of resistance of the section to tension and is designated Wyr; similarly, Jy/h2 is called the moment of resistance of the section to compression

and denote Wyc, so

and therefore

If the neutral axis is the axis of symmetry of the section, then h1 = h2 = h/2 and, therefore, Wyp = Wyc, so there is no need to distinguish them, and they use the same notation:

calling W y simply the moment of resistance of the section. Consequently, in the case of a section symmetrical about the neutral axis,

All the above conclusions were obtained on the basis of the assumption that the cross sections of the beam, when bent, remain flat and normal to its axis (hypothesis of flat sections). As has been shown, this assumption is valid only in the case when the extreme (end) sections of the beam remain flat during bending. On the other hand, from the hypothesis of plane sections it follows that elementary forces in such sections should be distributed according to a linear law. Therefore, for the validity of the resulting theory of flat pure bending, it is necessary that the bending moments at the ends of the beam be applied in the form of elementary forces distributed along the height of the section according to a linear law (Fig. 96), coinciding with the law of stress distribution along the height of the section beams. However, based on the Saint-Venant principle, it can be argued that changing the method of applying bending moments at the ends of the beam will cause only local deformations, the influence of which will affect only a certain distance from these ends (approximately equal to the height of the section). The sections located throughout the rest of the length of the beam will remain flat. Consequently, the stated theory of flat pure bending for any method of applying bending moments is valid only within the middle part of the length of the beam, located from its ends at distances approximately equal to the height of the section. From here it is clear that this theory is obviously inapplicable if the height of the section exceeds half the length or span of the beam.

Calculate bending beam There are several options:
1. Calculation of the maximum load that it will withstand
2. Selection of the section of this beam
3. Calculation based on maximum permissible stresses (for verification)
let's consider general principle for selecting a beam section on two supports loaded with a uniformly distributed load or concentrated force.
To begin with, you will need to find the point (section) at which there will be a maximum moment. This depends on whether the beam is supported or embedded. Below are diagrams of bending moments for the most common schemes.

After finding the bending moment, we must find the moment of resistance Wx of this section using the formula given in the table:

Further, when dividing the maximum bending moment by the moment of resistance in a given section, we get maximum stress in beam and we must compare this stress with the stress that our beam of a given material can generally withstand.

For plastic materials(steel, aluminum, etc.) the maximum voltage will be equal to material yield strength, A for fragile(cast iron) – tensile strength. We can find the yield strength and tensile strength from the tables below.

Let's look at a couple of examples:
1. [i] You want to check whether an I-beam No. 10 (steel St3sp5) 2 meters long, rigidly embedded in the wall, will support you if you hang on it. Let your mass be 90 kg.
First, we need to select a design scheme.

This diagram shows that the maximum moment will be at the seal, and since our I-beam has equal section along the entire length, then the maximum voltage will be in the termination. Let's find it:

P = m * g = 90 * 10 = 900 N = 0.9 kN

M = P * l = 0.9 kN * 2 m = 1.8 kN * m

Using the I-beam assortment table, we find the moment of resistance of I-beam No. 10.

It will be equal to 39.7 cm3. Let's convert it to cubic meters and get 0.0000397 m3.
Next, using the formula, we find the maximum stresses that arise in the beam.

b = M / W = 1.8 kN/m / 0.0000397 m3 = 45340 kN/m2 = 45.34 MPa

After we have found the maximum stress that occurs in the beam, we can compare it with the maximum permissible stress equal to the yield strength of steel St3sp5 - 245 MPa.

45.34 MPa is correct, which means this I-beam will withstand a mass of 90 kg.

2. [i] Since we have quite a large supply, we will solve the second problem, in which we will find the maximum possible mass that the same I-beam No. 10, 2 meters long, will support.
If we want to find the maximum mass, then we must equate the values ​​of the yield strength and the stress that will arise in the beam (b = 245 MPa = 245,000 kN*m2).